Math Strategies: Solving Problems Using Guess and Check
Welcome to the last post in my series on problem solving strategies! There are so many different ways to approach math word problems, but it’s important that we share these various methods with kids so that they can be equipped to tackle them. This week I’m explaining a strategy that doesn’t sound overly mathematical, but can be extremely useful when done properly: solving problems using guess and check! As with the other strategies I’ve discussed, it’s important to help kids understand how to use this method so that they are not randomly pulling answers out of their head and wasting time.

–>Pssst! Do your kids need help making sense of and solving word problems? You might like this set of editable word problem solving templates! Use these with any grade level, for any type of word problem:
Guess & Check Math Strategy:
You may hear the name of this strategy and think, “Guess? Isn’t the whole point of math instruction to teach kids to solve problems so that they’re no longer merely guessing??”
While it is certainly true that we don’t want kids to simply guess random answers for every math problem they ever encounter, there are instances when educated guesses are important, valid and useful.
For instance, learning and understanding how to accurately estimate is an important mathematical skill. A good estimate, however, is not just a random guess. It takes effort and logic to formulate an estimate that makes sense and is (hopefully) close to the correct answer. (For fun and easy estimation practice, try this Mummy Math activity!)
Similarly, solving problems using guess and check is a process that requires logic and an understanding of the question so that it can be done in a way that is organized and time saving.
So what does guess and check mean? To be more specific, this strategy should be called, “Guess, Check and Revise.”
The basic structure of the strategy looks like this:
- Form an educated guess
- Check your solution to see if it works and solves the problem
- If not, revise your guess based on whether it is too high or too low
This is a useful strategy when you’re given the total and you’re asked to find the kinds or number of things making up the total.
Or when the question asks for the value of two or more different kinds of things.
For instance, you might be asked how many girls and how many boys are in the class, or how many cats and how many dogs a pet owner has.
When guess and check seems like an appropriate strategy for a word problem, it will be helpful and necessary to then organize the information in a table or list to keep track of the different guesses.
This provides a visual of the important information, and will also help ensure that subsequent guesses are logical and not random.
Using the Guess and Check Strategy:
To begin, students should make a guess using what they know from the problem. This first guess can be anything at all, so long as it follows the criteria given. Then, once a guess is made, students can begin to make more educated guesses based on how close they are to the correct answer.
For example, if their initial guess gives a total that is too high, they need to choose smaller numbers for their next guess.
Likewise, if their guess gives a total that is too low, they need to choose larger numbers.
The most important thing for students to understand when using this method is that after their initial guess, they should work towards getting closer to the correct answer by making logical changes to their guess. They should not be choosing random numbers anymore!
Here’s an example to consider:
In Ms. Brown’s class, there are 24 students. There are 6 more girls than boys. How many boys and girls are there?
Because we know the class total (24), and we’re asked to find more than one value (number of boys and number of girls), we can solve this using the guess and check method.
To organize the question, we can form a table with boys, girls and the total. Because we know there are 6 more girls than boys, we can guess a number for the boys, and then calculate the girls and the total from there.

With an initial guess of 12 boys, we see that there would be 18 girls, giving a total class size of 30. The total, however, should only be 24, which means our guess was too high. Knowing this, the number of boys is revised and the total recalculated.
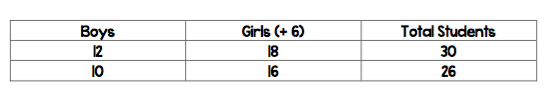
Lowering the number of boys to 10 would mean there are 16 girls, which gives a class total of 26. This is still just a little bit too high, so we can once again revise the guess to 9 boys. If there are 9 boys, that would mean there are 15 girls, which gives a class total of 24.
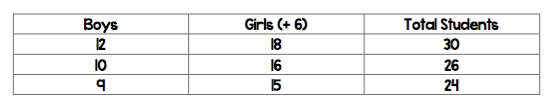
Therefore, the solution is 9 boys and 15 girls.
This is a fairly simple example, and likely you will have students who can solve this problem without writing out a table and forming multiple guesses. But for students who struggle with math, this problem may seem overwhelming and complicated.
By giving them a starting point and helping them learn to make more educated guesses, you can equip them to not only solve word problems, but feel more confident in tackling them.
This is also a good strategy because it helps kids see that it’s ok to make mistakes and that we shouldn’t expect to get the right answer on the first try, but rather, we should expect to make mistakes and use our mistake to learn and find the right answer.
What do you think? Do you use or teach this strategy to students? Do you find it helpful?

And of course, don’t miss the rest of the problem solving strategy series:
- Problem Solve by Solving an Easier Problem
- Problem Solve by Drawing a Picture
- Problem Solve by Working Backwards
- Problem Solve by Making a List
- Problem Solve by Finding a Pattern













My strategy was usually more “guess and hope for the best”. Yours sounds much wiser, lol! Thanks for sharing at the Thoughtful Spot!
Haha!! Yes, I think that’s what most people think of when they hear, “Guess and Check!” Hope this was helpful, 🙂
How exactly do you do this?
Sorry, your way was amazing…but I’m still confused:(
I’m so sorry you’re confused. Can you explain what part you don’t understand so I can try and make it clearer? The object is to make a reasonable guess, and then adjust your guess based on if your answer is too high or too low (try to be logical rather than random).
Nicely explained post and a different logic for solving problems “Guess and check”, I don’t know that how much helpful it is but I’m sure that your idea will help to change the thinking of readers. Thanks for sharing a different kind of idea for problem solving.